Need A Tutor?    |   Need Homework Help?                                                                             Help and Support     | Join or Cancel

What are the portfolio weights for a portfolio that has 185 shares of
Stock A that sell for $94 per share and 160 shares of
Stock B that sell for $122 per share?
(Do not round intermediate calculations and round your answers to 2 decimal places, e.g., 32.1616.)
 
Calculation of Weight of Stock A and Stock B

Portfolio	No. of shares	Market Price per Share	Total Market Value	Weight of Stock
Stock A	185 Shares	$94	$17,390	$17,390 / $36,910 = 0.4711
Stock B	160 Shares	$122	$19,520	$19,520 / $36,910 = 0.5289
TOTAL			$36,910	1.00

 

 
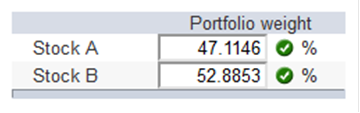
Explanation:
The portfolio weight of an asset is the total investment in that asset divided by the total portfolio value.
First, we will find the portfolio value, which is:
  
Total value = 185($94) + 160($122)
Total value = $36,910
  
The portfolio weight for each stock is:
  
x_A = 185($94) / $36,910
x_A = .4711 or 47.11
  
x_B = 160($122) / $36,910
x_B = .5289 or 52.89
   
A portfolio is invested 27 percent in Stock G, 42 percent in Stock J, and 31 percent in Stock K.
The expected returns on these stocks are 9.5 percent, 12 percent, and 17.4 percent, respectively.
  
What is the portfolio’s expected return?
(Do not round intermediate calculations and enter your answer as a percent rounded to 2 decimal places, e.g., 32.16.)
  

 
Expected return = [(27%*9.5) + (42%*12) + (31%*17.4)]
which is equal to
= 12.999 (13.00%
 
Explanation:
The portfolio's expected return is the sum of the weight (position) in each stock times the rate of return of each stock.
The expected portfolio's return is:
  
E(R) = .27(.095) + .42(.120) + .31(.174)
E(R) = .1300, or 13.00%
   
Consider the following information:
 

State of	Probability of			Rate of Return If State Occurs
Economy	State of Economy			Stock A			Stock B			Stock C
Boom		.20			.361			.461			.341
Good		.40			.131			.111			.181
Poor		.30			.021			.031		−	.067
Bust		.10		−	.121		−	.261		−	.101

 
Your portfolio is invested 31 percent each in A and C and 38 percent in B. What is the expected return of the portfolio?
(Do not round intermediate calculations and enter your answer as a percent rounded to 2 decimal places, e.g., 32.16.)
  
What is the variance of this portfolio?
(Do not round intermediate calculations and round your answer to 5 decimal places, e.g., 32.16161.)
 
What is the standard deviation of this portfolio?
(Do not round intermediate calculations and enter your answer as a percent rounded to 2 decimal places, e.g., 32.16.)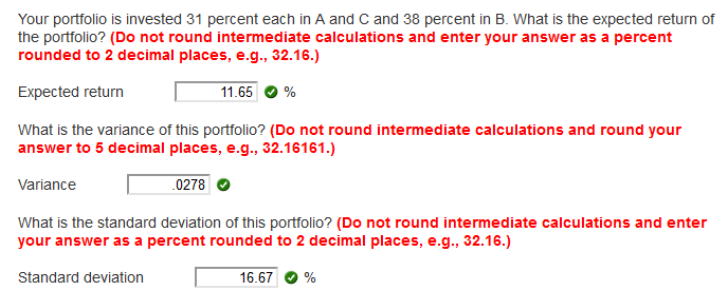
 

 
Explanation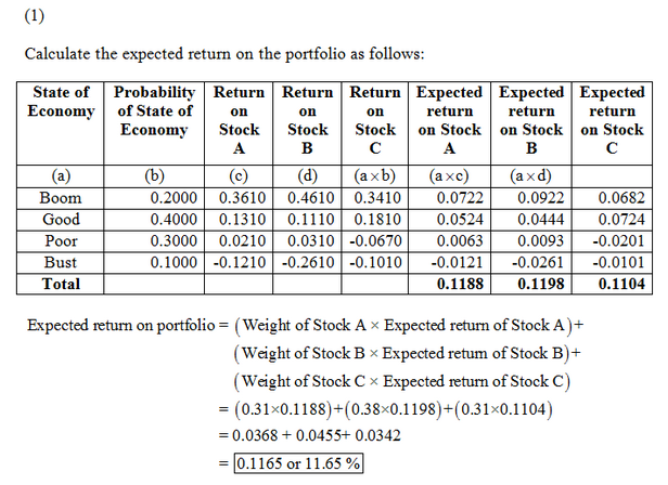


 
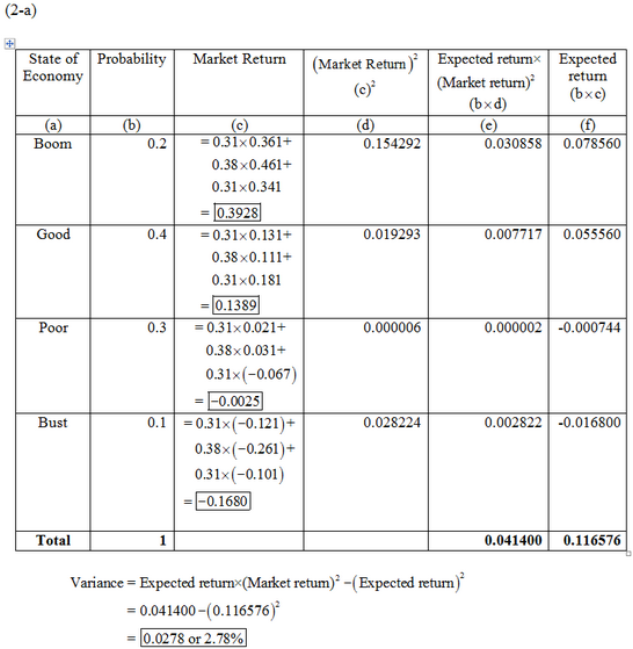
.041400 – (.116576) ^2 = .0278100362 (.0278)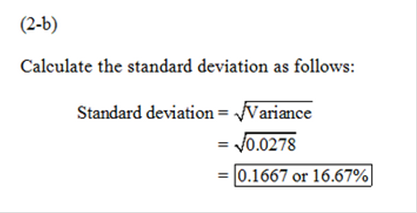
 

Explanation:
This portfolio does not have an equal weight in each asset. We first need to find the return of the portfolio in each state of the economy.
To do this, we will multiply the return of each asset by its portfolio weight and then sum the products to get the portfolio return in each
state of the economy. Doing so, we get:
 

Boom:	Rp	= .31(.361) + .38(.461) + .31(.341)
	Rp	= .3928, or 39.28%

 

Good:	Rp	= .31(.131) + .38(.111) + .31(.181)
	Rp	= .1389, or 13.89%

 

Poor:	Rp	= .31(.021) + .38(.031) + .31(–.067)
	Rp	= –.0025 or –.25%

 

Bust:	Rp	= .31(–.121) + .38(–.261) + .31(–.101)
	Rp	= –.1680, or –16.80%

 
And the expected return of the portfolio is:
 
E(R_p) = .20(.3928) + .40(.1389) + .30(–.0025) + .10(–.1680)
E(R_p) = .1166, or 11.66%
 
To calculate the standard deviation, we first need to calculate the variance.
To find the variance, we find the squared deviations from the expected return.
We then multiply each possible squared deviation by its probability, and then sum.
The result is the variance. So, the variance and standard deviation of the portfolio is:
 
σ_p² = .20(.3928 – .1166)² + .40(.1389 – .1166)² + .30(–.0025 – .1166)² + .10(–.1680 – .1166)²
σ_p² = .02781
 
σ_p = .02781^1/2
σ_p = .1668, or 16.68%
   
A stock has a beta of 1.14, the expected return on the market is 10.8 percent, and the risk-free rate is 4.55 percent.
 
What must the expected return on this stock be?
(Do not round intermediate calculations and enter your answer as a percent rounded to 2 decimal places, e.g., 32.16.)
 
Expected return             %

4.55 + 1.14 (10.8 - 4.55) = 11.68 %
 
Explanation:
The CAPM states the relationship between the risk of an asset and its expected return. The CAPM is:
 
E(R_i) = R_f + [E(R_M) – R_f] × β_i
 
Substituting the values we are given, we find:
 
E(R_i) = .0455 + (.1080 – .0455)(1.14)
E(R_i) = .1168, or 11.68%
   
Consider the following information on a portfolio of three stocks:
 

State of	Probability of			Stock A			Stock B			Stock C
Economy	State of Economy			Rate of Return			Rate of Return			Rate of Return
Boom		.12			.07			.32			.45
Normal		.55			.15			.27			.25
Bust		.33			.16		−	.26		−	.35

 
a. If your portfolio is invested 40 percent each in A and B and 20 percent in C,
what is the portfolio’s expected return, the variance, and the standard deviation?
(Do not round intermediate calculations. Round your variance answer to 5 decimal places, e.g., 32.16161.
Enter your other answers as a percent rounded to 2 decimal places, e.g., 32.16.)
 
b. If the expected T-bill rate is 4.5 percent, what is the expected risk premium on the portfolio?
(Do not round intermediate calculations and enter your answer as a percent rounded to 2 decimal places, e.g., 32.16.)
 
Expected risk premium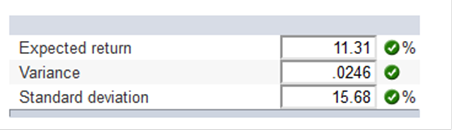  
 

 

 
 
Explanation:
a. 
We need to find the return of the portfolio in each state of the economy.
To do this, we will multiply the return of each asset by its portfolio weight and then sum the products to get
the portfolio return in each state of the economy. Doing so, we get:
 

Boom:	Rp	= .40(.07) + .40(.32) + .20(.45)
	Rp	= .2460, or 24.60%

 

Normal:	Rp	= .40(.15) + .40(.27) + .20(.25)
	Rp	= .2180, or 21.80%

 

Bust:	Rp	= .40(.16) + .40(–.26) + .20(–.35)
	Rp	= –.1100, or –11.00%

 And the expected return of the portfolio is:
 
E(R_p) = .12(.2460) + .55(.2180) + .33(–.1100)
E(R_p) = .1131, or 11.31%
 
To calculate the standard deviation, we first need to calculate the variance.
To find the variance, we find the squared deviations from the expected return.
We then multiply each possible squared deviation by its probability, and then sum.
The result is the variance. So, the variance and standard deviation of the portfolio are:
 
σ²_p = .12(.2460 – .1131)² + .55(.2180 – .1131)² + .33(–.1100 – .1131)²
σ²_p = .02460
 
σ_p = .02460^1/2
σ_p = .1568, or 15.68%
 
b.
The risk premium is the return of a risky asset, minus the risk-free rate. T-bills are often used as the risk-free rate, so:
 
RP_i = E(R_p) – R_f
RP_i = .1131 – .0450
RP_i = .0681, or 6.81%
   
What is the beta of the following portfolio?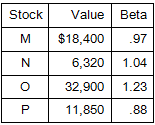 
 

 
.99
1.11
1.15
1.08
1.04
 

	Value	% weight	Beta
M	18400	26.49%	0.97
N	6320	9.10%	1.04
O	32900	47.36%	1.23
P	11850	17.06%	0.88
Total	69470	100.00%	1.084

Calculate the weight of each stock in the portfolio, Value / Total Portfolio Value.
Now, Multiply each beta with its equivalent weight in the portfolio and sum it up.
Portfolio beta = 0.97 x 26% + 1.04 x 9% + 1.23 x 47% + 0.88 x 17% = 1.084
   
A stock has a beta of 1.48 and an expected return of 17.3 percent.
A risk-free asset currently earns 4.6 percent.
If a portfolio of the two assets has a beta of .98, then the weight of the stock must be ___ and the risk-free weight must be___.
 
.72; .28
.56; .44
.66; .34
.34; .66
.44; .56
 
Calculation of weight of the stock and weight of the risk free asset
stock expected return = 17.3%
stock beta value = 1.48
risk free asset beta value is = 0
risk free asset return = 4.6
portfolio beta is = 0.98
let taken weight of the stock is X
so weight of the risk free asset is = 1-X
portfolio beta = stock weight*beta+riskfree weight*beta
0.98 = X*1.48+(1-X)*0
0.98= 1.48X+0
1.48X= 0.98
X = 0.66
66%
weight of the risk free asset is = 1-0.66
= 0.34
= 34%
   
You would like to create a portfolio that is equally invested in a risk-free asset and two stocks.
One stock has a beta of 1.39. What does the beta of the second stock have to be if you want the portfolio to be equally as risky as the overall market?
 
1.23
1.61
1.55
.97
.72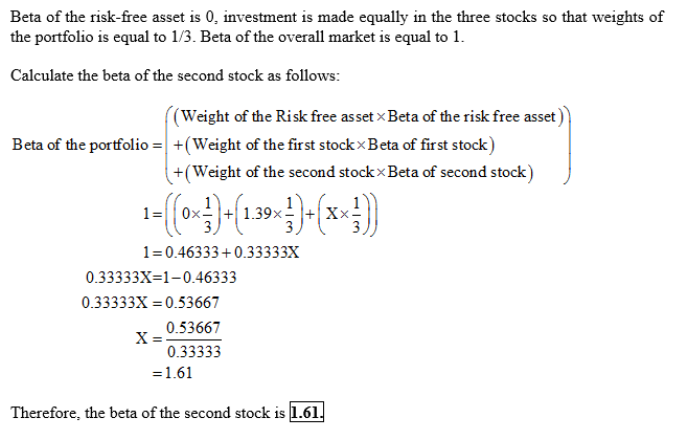
 
 
You own a $58,600 portfolio comprised of four stocks.
The values of Stocks A, B, and C are $11,200, $17,400, and $20,400, respectively.
What is the portfolio weight of Stock D?
 
12.58 percent
16.38 percent
10.33 percent
12.10 percent
15.39 percent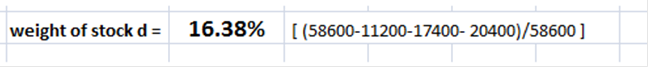
 
 
Stock A has an expected return of 14.4 percent and a beta of 1.21.
Stock B has an expected return of 12.87 percent and a beta of 1.06.
Both stocks have the same reward-to-risk ratio. What is the risk-free rate?
 
1.79 percent
2.28 percent
3.35 percent
2.06 percent
1.92 percent
 
 
A stock has a beta of 1.32 and an expected return of 12.8 percent.
The risk-free rate is 3.6 percent. What is the slope of the security market line?
 
6.97 percent

Slope = (.128 -.036)/1.32 = .0697, or 6.97 percent
   
Stock J has a beta of 1.52 and an expected return of 15.76percent.
Stock K has a beta of .98 and an expected return of 11.44 percent.
What is the risk-free rate if these securities both plot on the security market line?
 
3.60 percent

(.1576-Rf)/1.52 = (.1144 -Rf)/.98
Rf = .0360, or 3.60 percent
   
Bama Entertainment has common stock with a beta of 1.22.
The market risk premium is 8.1 percent and the risk-free rate is 3.9 percent.
What is the expected return on this stock?
 
13.78 percent

E(R) = .039 + 1.22(.081) = .1378, or 13.78 percent
   
BJB stock has an expected return of 17.82 percent.
The risk-free rate is 4.6 percent and the market risk premium is 8.2 percent. What is the stock's beta?
 
1.61

E(R) = .1782 = .046 + β(.082)
β = 1.61
   
You own a stock that has an expected return of 15.72 percent and a beta of 1.33.
The U.S. Treasury bill is yielding 3.82 percent and the inflation rate is 2.95 percent. What is the expected rate of return on the market?
 
12.77 percent

E(R) = .1572 = .0382 + 1.33 (Rm -.0382)
Rm = .1277, or 12.77 percent
   
A stock has a beta of 1.10, an expected return of 12.11 percent, and lies on the security market line.
A risk-free asset is yielding 3.2 percent.
You want to create a portfolio valued at $12,000 consisting of Stock A and the risk-free security such that the portfolio beta is .80.
What rate of return should you expect to earn on your portfolio?
 
9.68 percent

E(R) = .1211= .032 + 1.10(MRP)
MRP = .0810
E(RP) = .032 + .80(.0810) = .0968, or 9.68 percent
   
You own a portfolio that has $2,200 invested in Stock A and $1,300 invested in Stock B.
If the expected returns on these stocks are 11 percent and 17 percent, respectively, what is the expected return on the portfolio?
 
13.23 percent

E(R) = [$2,200 / ($2,200 + 1,300)] ×.11 + [$1,300 / ($2,200 + 1,300)] ×.17 = .1323, or 13.23 percent
   
You own a portfolio equally invested in a risk-free asset and two stocks.
If one of the stocks has a beta of 1.86 and the total portfolio is equally as risky as the market,
what must the beta be for the other stock in your portfolio?
 
1.14

βP = 1 = (1 / 3)(0) + (1 / 3) (1.86) + (1 / 3) (x)
x = 1.14
   
A stock has a beta of 1.32, the expected return on the market is 12.72, and the risk-free rate is 4.05.
What must the expected return on this stock be?
 
15.49 percent

E(R) = .0405 + 1.32(.1272 -.0405) = .1549, or 15.49 percent
   
A stock has an expected return of 14.3 percent, the risk-free rate is 3.9 percent, and the market risk premium is 7.8 percent.
What must the beta of this stock be?
 
1.33

E(R) = .143 = .039 + β (.078)
β = 1.33
   
A stock has a beta of 1.48 and an expected return of 17.3 percent.
A risk-free asset currently earns 4.6 percent.
If a portfolio of the two assets has a beta of .98, then the weight of the stock must be ___ and the risk-free weight must be___.
 
.66; .34
βP = .98 = x(1.48) + (1 -x)(0)
x = .66


Stock weight is .66 and the risk-free weight is .34
Stock Y has a beta of 1.28 and an expected return of 13.7 percent.
Stock Z has a beta of 1.02 and an expected return of 11.4 percent.
What would the risk-free rate have to be for the two stocks to be correctly priced relative to each other?
 
2.38 percent

(.137 -Rf)/1.28 = (.114 -Rf)/1.02
Rf = .0238, or 2.38 percent
   
Stock J has a beta of 1.06 and an expected return of 12.3 percent, while Stock K has a beta of .74 and an expected return of 6.7 percent.
If you create portfolio with the same risk as the market, what rate of return should you expect to earn?
 
11.25 percent

βP = 1.0 = 1.06x+ .74 (1 -x)
x = .8125
E(RP) = .8125 (.123) + (1 -.8125) (.067) = .1125, or 11.25 percent