|
Accounting | Business | Computer Science | General Studies | Math | Sciences | Civics Exam | Help/Support | Join/Cancel | Contact Us | Login/Log Out Intermediate
Algebra Chapter 1 2 3 4 5 6 7 8 9 10 11
Elementary and Intermediate Algebra
Chapter 5: Factoring
These Study Guides Are 100% Free
 Donate Please (Help keep it free)! This course is 100% Free. If you need more help with your class, I suggest Joining Now! Give the prime factorization for the integer 66. 2 · 3 · 11 66 can be written as 2 · 33 33 can be written as 3 · 11 So, 2 · 3 · 11 = 66 Give the prime factorization for the integer 236. 22 · 59 236 can be written as 2 · 118 118 can be written as 2 · 59 So, 2 · 2 (22) · 59 = 236 Find the greatest common factor for the group 48, 80. 16 We will find the GCF using repeated division. 48 80 2 24 40 2 12 20 2 6 10 2 3 5 There are no more common factors, so we can now find The greatest common factor by multiplication. 2 · 2 · 2 · 2 = 16 Find the greatest common factor for the group 42, 66, 78. 6 2 42 66 78 2 21 33 39 3 7 11 13 There are no more common factors, so we can now find the greatest common factor by multiplication. 2 · 2 · 3 = 12 However, 12 is not a common factor for each number in the group, So, we need to reduce. 2 · 3 = 6 6 is the only common factor in the group, so 6 is the greatest common factor for the group. Find the greatest common factor for the group 6y2, 15y3. 3y2 Since we are looking for the greatest common factor (GCF) with Variables (y), the variable is an unknow number, so, we cannot use the same approach as above. We will simply factor both expressions and determine the GCF. 6y2 = 2 · 3 · y · y 15y3 = 3 · 5 · y · y · y The GCF in this case will be 3 and y · y So, the answer = 3 · y · y or 3y2 Find the greatest common factor for the group 12a2b, 18ab2, 24a3b3. 6ab The same as above. We will need to factor each expression. 12a2b = 3 · 2 · 2 · a · a · b 18ab2 = 3 · 3 · 2 · a · b · b 24a3b3 = 3 · 2 · 2 · 2 · a · a · a · b · b · b Remember, the GCF needs to be common. 2 · 3 is a far as we can go, so we need to use 2 · 3 as the coefficient. Two of the expressions contain only one a and one b (12a2b and 18ab2) Therefore, the GCF is 2 · 3 · a · b or 6ab. Factor the polynomial 5x2 – 10x completely. 5x (x – 2) First, we know that x2 = xx (when variables sit next to each other, that means multiplication) When we are told to factor a polynomial, we need to split the polynomial using common terms if we can. In this case, we know that 5x (2) = 10x and 5x (x) = 5x2. 5x is the common term, so now we can factor. We start with 5x 5x(x) = 5x2 and 5x (-2) = -10x The answer is 5x (x – 2) Factor the polynomial 6x2y2 + 12xy2 + 12y2 completely. 6y2(x2 + 2x + 2) If we look carefully, we can see 6y2 is the Common Term. (12y2 does not contain x) Now we divide each expression by 6y2. 6x2y2 / 6y2 = x2 12xy2 / 6y2 = 2x 12y2 / 6y2 = 2 Now we put the equation together in proper order. (remember 6y2 is the common factor) Answer = 6y2(x2 + 2x + 2) Factor the polynomial 3a3b – 3ab3 completely. 3ab (a – b) (a + b) Explanation: Factor out common term 3ab(a2 – b2) a2 – b2: (a + b) (a – b) 3ab (a + b) (a – b) Factor the polynomial a2 + 2a – 24. (a + 6) (a – 4) First, we would simplify a2. a · a = a2 = we have the first part to the answer. > (a)(a) Since we know we need to factor -24 by division, and the 2a is positive, we need to find two numbers which equal -24, and +2a. We can write them all out just this time, so we understand what we are looking for. I know it is annoying, however, it is best to do this until you understand better. 1 · 24 = 24 2 · 12 = 24 3 · 8 = 24 4 · 6 = 24 Now we look for the set which equals +2a. It is simple, 6a – 4a = 2a, so we now have the solution. Remember, we found the first part to the answer (a)(a) because that equals a2. Now we simply add the last part which we just found. (a + 6) (a - 4) We can check our answer (just once since you can check the rest on your own). (a · a) + (-4 · a) + (6 · a) + (6 · -4) a2 - 4a + 6a – 24 a2 (-4a + 6a) -24 a2 + 2a - 24 Factor the polynomial 4b2 – 28b + 49 completely. (2b – 7)2 Rewrite 4b2 as (2b)2 leaves us with (2b)2 – 28b + 49 Now we rewrite 49 as 72 leaves us with (2b)2 -28b + 72 Now we check the middle term which is 2 times 7 which is being squared. 2 · 2b · 7 = 28b Now we rewrite the polynomial. (2b – 7)2 Factor the polynomial 3m3 + 27m completely. 3m(m2 + 9) I am going to explain quickly because we have been over this already. 3m will be the GCF in this case. Now we simply divide: 3m3 + 27m / 3m. 3m3 / 3m = m2 27m / 3m = 9 Now we simply write the equation. 3m (m2 + 9) Factor the polynomial ax – ay + bx - by completely. (a + b) (x – y) First, we factor out the GCF (greatest common factor) from each group. a (x – 1y) + b (x – 1y) Then we factor the polynomial by factoring out the GCF. (x – 1y) (a + b) Rewrite (a + b) (x – y) Factor the polynomial ax – 2a -5x + 10 completely. (a – 5)(x – 2)  Factor the polynomial 6b2 – 7b - 5 completely. (3b – 5)(2b + 1)  Factor the polynomial m2 + 4mn + 4n2 completely. (m + 2n)2 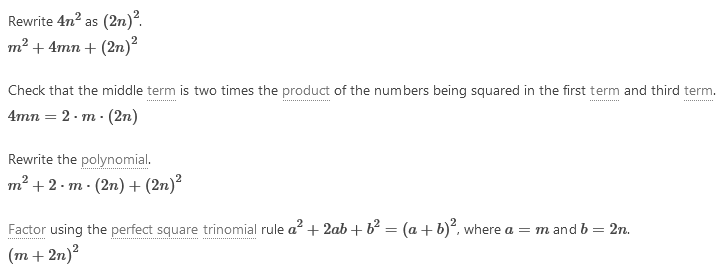
Factor the polynomial 2a2 – 13a + 15 completely.
(2a – 3) (a – 5) We have had a similar problem before. We need to factor 2a2 first = (2a) (a). Now we need to find two numbers which = 15, and -13a Since 13a will be negative, we know that the divisors of 15 must be negative. (-3) (-5) = 15 We have the solution. (2a) (a) + (-3) (-5) The answer is (2a – 3) (a – 5) z (z + 3) (z + 6) This is easier than it looks. All we need to do is think of 2 numbers that equals 18 (by multiplication) and 9 (by addition or subtraction). There are no negatives, so they must be positive. Once you get good at this, You can simply CHECK the most logical multiple-choice answer and cut through all of this. It is highly recommended that you CHECK EVERY ANSWER ANYWAY! That is what I did whenever I could, and I graduated with high honors (2 degrees, working on my 3rd). You will want to take the shortest and easiest route on any problems you can, so you will have more time for the truly difficult problems! This will be the same procedure as above. We want to breakdown or factor z3 first. (z)(z)(z) = z3 so, we know the first part is z(z)(z) Now we find the numbers that equal 18 (by multiplication) and 9 (by addition or subtraction). It is rather simple. 6 + 3 = 9 6 · 3 = 18 z(z) + (3) (z) + (6) The answer is z (z + 3) (z + 6). Factor the polynomial x3 + 125 completely. (x + 5) (x2 – 5x + 25) This is easier than it looks also. Whenever you see an equation like this, You know x2 and x have been cancelled out. First, we do as we have been doing. We factor x3 first. (x) (x2) = x3, so we have the first part. We need to find two numbers that = 125 by multiplication, and that would be (5)(25). We have the first part of the equation (x + 5) and part of the 2nd part, (x2 + 25). We need to cancel out “x2 and x” and the logical way to do so is add a “-x” to the second part of the equation. It needs to be (-5 and an x) because the first part is (x + 5). The answer is (x + 5) (x2 – 5x + 25). Like I said before, use some logic with problems which are multiple choice, And CHECK the most logical multiple-choice answer, because you really should check the answer you select anyway! Factor the polynomial a4 – ab3 completely. a(a – b)(a2 + ab + b2) 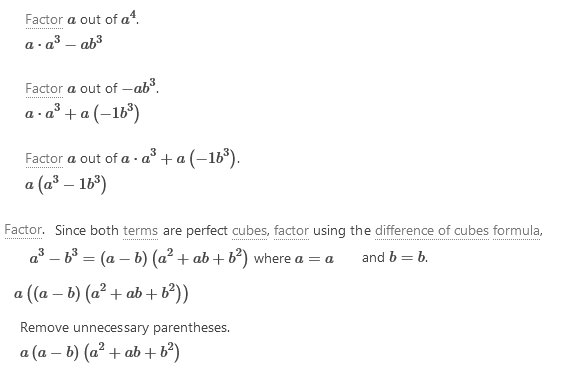 Solve the equation x2 + 6x + 9 = 0. -3 We would use the “perfect square rule” for this. (x+3)2 = 0 Set the x + 3 equal to 0. x + 3 = 0 Subtract 3 from both sides of the equation. x = −3 Solve the equation 2x2 + 5x - 12 = 0 Factor by grouping. (2x − 3) (x + 4) = 0 2x – 3 = 0 and x + 4 = 0 First, we solve 2x – 3 = 0 Add 3 to each side. 2x = 3 Then we divide each side by 2 x = Next, we solve x + 4 = 0 Subtract 4 from each side x = -4 Solve the equation 3x3 = 12x. 0, -2, 2 First, we subtract 12x from both sides of the equation. 3x3 - 12x = 0 Now we factor the polynomial like we have done before 3x (x + 2) (x − 2) = 0 If any individual factor on the left side of the equation is equal to 0, the entire expression will be equal to 0 These are the expressions we need to solve. We will not solve for 3x, because x will = 0. However, we will represent x = 0 as the first answer. x = 0 Next we solve x + 2 = 0, so we subtract 2 from each side. x = -2 Next, we will solve x − 2 = 0, so we add 2 to both sides. x = 2 That’s how we get the answer/s x = 0 x = -2 x = 2 Solve the equation (2x – 1) (3x + 5) = 5 -2, First, let’s simplify! 2x · 3x = 6x2 2x · 5 = 10x -1 · 3x = -3x -1 · 5 = -5 6x2 + 10x – 3x – 5 = 5 6x2 + 7x – 5 = 5 Now we will subtract 5 from each side and solve for 0. 6x2 + 3x – 5 - 5 = 0 Simplify once again 6x2 + 3x – 10 = 0 Now Factor by grouping. (6x − 5) (x + 2) = 0 If any individual factor on the left side of the equation is equal to 0, the entire expression will be equal to 0. Factor 6x – 5 = 0 we add 5 to both sides, then divide both sides by 6 x = Factor x + 2 = 0 we subtract 2 to both sides. x = -2 Solve the equation 2, 4 First, we need to move the value of x to the top of the fractions… Next, we will multiple the terms on the left side by 8 to eliminate the fractions… x2 – 6x + 8 = 0 Next, we factor using the AC Method (x − 4) (x − 2) = 0 If any individual factor on the left side of the equation is equal to 0, the entire expression will be equal to 0. x – 4 = 0 (add 4 to both sides) x = 4 x − 2 = 0 (add 2 to both sides) x = 2 Write a solution for the following. If the length of a rectangle is 3 feet longer than the width and the diagonal is 15 feet, then what are the length and width? Length 12 ft, width 9 ft Let w = width of rectangle, then w + 3 = length Pythagorean theorem: w2 + (w + 3)2 = 152 w2+ (w + 3) (w + 3) = 152 w2 + (w2 + 3w + 3w + 9) = 225 w2 + w2 + 6w + 9 = 225 2w2 + 6w + 9 = 225 2w2 +6w - 216 = 0 w2 + 3w - 108 = 0 Now we factor (w - 9) (w + 12) = 0 w – 9 = 0 (add 9 to both sides) Width = 9 w + 12 = 0 (subtract 12 from both sides) -12 Since w + 3 = length, 9 + 3 = 12 length = 12 The sum of two numbers is 4, and their product is -32. Find the numbers. -4 and 8 x (x - 4) = -32 x2 – 4x = -32 x2 – 4x + 32 = 0 (x + 4) (x - 8) = 0 x + 4 = 0 (subtract 4 from both sides) x = -4 x - 8 = 0 (add 8 to both sides) x = 8 A ball dropped from a height of 64 feet. Its height above the earth in feet is given, h(t) = 16t2 + 64, where t is the number of seconds after it dropped. a. Find h(1) 48 feet Substitute t with 1 and solve: h (1) = -16(1)2 + 64 h (1) = -16(1) + 64 h (1) = -16 + 64 h (1) = 48 feet b. How does it take for the ball to fall to earth? 2 seconds Simply substitute h(t) with a 0 and solve for t height when it falls to earth. 0 = -16t2 + 64 16t2 = 64 t2 = 64/16 t2 = 4 t = 2 seconds |
| Home |
Accounting & Finance | Business |
Computer Science | General Studies | Math | Sciences |
Civics Exam |
Everything
Else |
Help & Support |
Join/Cancel |
Contact Us |
Login / Log Out |